Microsteps - Mega Benefits
Here's a little quiz to test your knowledge on step motors: Microstepping is more accurate than fullstep control - true or false? If you answered "true," you might be surprised.
In a step motor, perfectly spaced states divide the electrical cycle into an integral number of equal intervals. If the rotor followed the excitation signal with perfectly spaced incremental moves, microstepping would be, without question, more accurate than full-step. But step motors are not perfect. No matter how finely you articulate the electrical cycle, there will be some amount of error.
Despite these errors, microstepping, as a control technique, is smoother and quieter than full or half-step. And, with some intervention that improves microstep accuracy while adding little or no cost, it can live up to all its capabilities.
Error sources
Two motor features are at the root of the problem: nonlinear constituent materials and a pronounced periodic structure. The nonlinearities come from magnetic hysteresis and variations in permeability. These two phenomena cause the magnetic induction to deviate from true proportionality with winding currents.
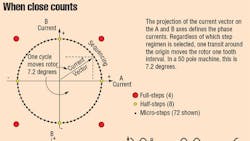
Cyclic error is caused by a reluctance torque, independent of electrical excitation, that develops between the rotor and stator teeth. There are four cycles of such error in the shaft output corresponding to interactions between the leading and lagging edges of the rotor teeth and the N and S sides of the pole face. Thus, with each 1/4 of a tooth interval, the rotor will first lag and then lead the anticipated perfect positions. This error repeats four times, or cycles, per tooth.
Actual performance varies markedly among manufacturers and designs. Motors most suited to microstepping exhibit approximately ± 3 arc-min (0.05°) of cyclic error. Other designs register up to ± 20 arcmin. At a resolution of 25,000 steps, 20 arc-min amounts to 23 microsteps.
Full-stepping is more accurate than microstepping because it uses positions that fall precisely on the zero-crossings of the cyclic error. Consequently, errors can be as small as 10 arc-sec, close to 60 times smaller than those found in microstepping. But, the quantization noise in a coarse, 1.8° stepping regimen is about 20 times larger than the cyclic error displayed by a good microstepping system. Coarse quantization noise in full-stepping eclipses cyclic error sustained in microstepping.
Combating cyclic error
Since cyclic error is repeatable, it's possible to anticipate its effect and compensate by distorting the waveform template. Some manufacturers provide selectable waveforms which, in effect, advance or retard the commanded position in opposition to the intrinsic error of the motor.
This technique can improve accuracy performance to ± 1 arc minute. However, the entire process depends on knowing the cyclic error and being able to synchronize the distortion.
Another way to reduce cyclic error is to modify the motor tooth design. One design slightly skews (twists) the rotor teeth along the shaft. This cancels the error and lets the machine operate with undistorted sinusoidal winding currents.
A more recent design modifies the spacing of stator teeth from 7.2° to 7.5.° The rotor teeth remain at 7.2.° This later approach nulls the cyclic error and is less costly than rotor skewing; in fact, it is essentially free.
Each method reduces the idle detent commonly felt in the de-energized motor. Each also reduces the holding torque by 4 or 5%. But the trade off is generally worth the improved accuracy and smoothness at slow speed.
In most cases, you can also reduce cyclic error by backing off a few percent from the manufacturer's recommended motor current. This, of course, sacrifices some torque, but it brings the core materials out of saturation and leads to smoother operation.
Blame the drive
Step-motor drives must accurately produce the required microstepping currents. The ideal current waves are in temporal quadrature (the A and B pulses) during movement and display half-wave symmetry. Any deviation is erroneous and will have some harmful effect depending on the character and severity of the distortion. Defects can produce significant, often severe, vibration at low speed, and cyclic-position error at rest.
Drive induced errors fall into six major categories: offset, crossover distortion, diagonal clipping, phase balance, current loop instability, and quantization noise.
Offset. Offset is simply the unintended introduction of a dc component to either or both phase currents. In the electrical state diagram the phase-current pattern appears displaced from center.
Offset produces cyclic error and torque ripple in synchronism with the electrical pole rate – 50 cycles/revolution. An offset of 1% causes a cyclic error of approximately 1 microstep at 25,000 steps/rev. Some drive manufacturers provide fine adjustments to precisely null output offsets.
Crossover distortion. Deadband is the most common crossover distortion. It occurs when an amplifier cannot produce an output for current near zero. The cause is usually a PWM scheme known as cycle-by-cycle current limiting.
The drive's current feedback monitor misinterprets switching transients as motor current and terminates the PWM cycle prematurely. The drives can induce rather severe cyclic error at four times the pole rate (200 steps/rev) characterized by a distinct, raspy "buzz" from the motor at low speeds.
Although cycle-by-cycle current limiting is easy to implement and uses few parts, it is more suitable for full or half-step drives than high precision microstepping.
Diagonal clipping. This occurs when an amplifier fails to recognize that output current is not falling at the same rate as the input command. The circuitry generally "discovers" its error at crossover. The result is cyclic error at four times the pole rate.
This effect often plagues cycle-bycycle schemes. It does not affect accuracy at rest. During motion, however, the waveform distortion causes acoustic noise and severe vibration. More sophisticated current monitoring and control methods completely remedy this problem.
Phase gain. The ideal waveforms (the A and B pulses), of course, have the same amplitude. If they don't, then the motor will develop cyclic error at twice the pole rate (100 steps/rev). Precision drives provide trim potentiometers to ensure balanced amplitude.
Amplifier instability. A PWM power amplifier is a sampled-data, feedback system, typically operating at about 20 kHz. Because its sampled data, the control loop may enter into a disturbed state known as limit cycle. Here, a limited-amplitude nuisance oscillation superpositions itself on the intended output, and the amplifier appears to hunt for the proper output.
A related problem is the intrusion of a sampling alias into the baseband. This results in a sustained oscillation at a submultiple of the PWM frequency.
Such activities may add to motor heating and produce irritating acoustic noise. They may also disturb rotor position.
Quantization noise. This is the error introduced when a continuous variable is digitized into a limitedprecision number. Microstepping drives maintain waveform templates (sines and cosines) in memory, which are usually stored in byte-wide format. This provides ±127 values with which to form the sinusiodal shapes.
Using more table entries to add resolution eventually results in a quantization error larger than the intended step size. The number format then needs more bits to avoid missing or overlapping states in the template. In overlapping, there are many points around the electrical state diagram where a step simply does not move the vector. In other regions the vector may move slightly or inordinately far. The microsteps are effectively lost in the quantization noise.
Though several drives behave this way, they'd still be adequate if the engineer obtained the necessary articulation. Eight-bit arithmetic successfully renders no more than 36,000 steps/rev without overlapping states.
Sticky situation
A load is a passive object waiting for the motion system to position it correctly. Certain attributes of some loads make the control problem difficult or intractable. One such phenomenon is static friction; sometimes referred to whimsically as "stick-tion."
When a current vector is established in the step-motor windings, the rotor undergoes a capture transient that moves it into the center of a potential well. The action is analogous to a marble settling into a valley on a wavy surface. The depth of the well is expressed in units of potential energy.
Just as with the marble, the rotor is not actually locked in equilibrium at the bottom of the well, but rather, rests there until an external influence displaces it. Once displaced, the rotor undergoes a restoring influence exerted by the walls of the well. This is the operating detent (OD). It is a torque wave with amplitude, Tmax, and the same spatial frequency as the rotor teeth. Its existence depends on the presence of a current vector in the machine's windings. If the vector is removed (zeroed), the OD vanishes and the rotor can freely turn.
When the current vector is stationary, the OD is fixed. When the vector moves (current cycling), the OD also moves; doing so in rather precise spatial synchronism with the phase of the vector.
Running on error. The negativeslope, zero crossing of the detent wave represents the commanded position where the rotor tends to rest in the absence of external torque. When the rotor accelerates its load, or moves against friction, or opposes gravity, the machine must produce torque to overcome these effects. It cannot produce torque at the zero crossing. It must set back or forward in the OD wave to sustain torque production.
This is what is known as "running on error." Whenever the step motor must hold position while producing torque, the rotor will not be at the commanded position. Furthermore, if torque greater than the value Tmax is needed, the rotor will slip over the crest of the OD wave, marking the onset of loss of synchronism, or stall.
Stick-tion and step size. Static friction arises from Coulomb forces between contacting surfaces. It generally is significantly more than the moving friction between the objects. And it appears abruptly at or near rest; tending to seize the two members together.
Once at rest, it will develop force (or torque) to a point, holding the surfaces stationary against other forces. Once it reaches a certain level of torque, though, the surfaces begin to move. Other forces can then overwhelm the moving friction and cause abrupt acceleration.
This "stickiness" imposes a minimum move size. After the rotor breaks free, it may fly past the commanded position; coming to rest beyond the intended location. Again, a minimum move is needed before any more motion occurs. In this way, static friction limits resolution regardless of the resolution of the drive.
Static friction plagues many applications including servo systems. If it degrades performance below specifications, then the only solutions may be to eliminate it with lubrication or gear down the transmission path between motor and load.
Not so fast
As the drive turns the step motor faster, the individual steps are executed at higher frequency. So, the amplifiers filter out the minute details by smoothing the sinusiodal current waveforms.
However, at higher speeds, the amplifiers saturate. They can no longer produce enough voltage to sustain high-frequency currents against the increasing reactance of the motor windings. The result is that the drive furnishes nearly square-wave voltages which produce essentially sawtooth winding currents. In these crude approximations of the desired sinewaves, the operating detent is still present and the rotor remains captive within it.
Once the motor slows to rest, the precise microstepping current vector comes back for high resolution positioning at the end of the move.
Full-stepping and microstepping drivers behave in nearly identical fashion when the amplifier saturates at high speed. Both drives impose squarewaves of voltage at the electrical pole rate and produce sawtooths of current in the windings. This is why the two technologies sound the same at high speed. At low speeds and rest, the operations diverge dramatically, however.
Microstepping also reduces motor heating. Sinusoidal waveforms have 70% as much root-mean-square (rms) content as square waves of the same amplitude. The ohmic heating effect of a current is proportional to rms squared. So, the sinusoids of microstepping produce approximately half as much winding heat as fullstep square waves.
At high speed, where the amplifiers saturate, the two techniques produce nearly identical currents and essentially the same heating.
Spring effect
Resonance is often the most poorly understood aspect of step motor operation. It is a commonly held belief that there are many resonances in a step motor, however, there is only one. It is also widely held that such techniques as microstepping, skewing of the rotor, or increasing the phase count will reduce or eliminate resonance. In fact, none of these approaches has any effect on damping resonance. What they really do is reduce resonance excitation by carefully tiptoeing around it. But, the resonance is still there; waiting to be excited by the inevitable gross move dynamics, such as acceleration and deceleration, inherent in a sequence.
Recall the restoring influence, discussed earlier, where the rotor tends to rest at the negative-slope, zerocrossing of the operating detent. There are fifty such crossings in one revolution; the spatial frequency being set by the fifty rotor teeth. The rotor is captive in the vicinity of one of these equilibrium points and remains attached as long as it's not asked to produce more than maximum torque.
The restoring influence, however, acts like a "torsion spring." It's a stretchy magnetic field between the stator and rotor teeth. When the rotor moves out of equilibrium, for example, as long as there's a change in the angle of the current vector, the restoring influence generates a torque proportional to the amount of displacement. So, as a first approximation, the dynamics of the step motor rotor are those of an inertial load being pulled on the end of a spring.
Thus, no matter how well designed the motor, no matter how finely it steps through the electrical cycle, gross moves such as acceleration/ deceleration transients, can excite severe ringing. The activity may build from one event to the next with successive transient responses. This is why you are often told to plan on using only 50% of the motor's available torque. The machine will use the other 50% to vibrate around equilibrium.
A more serious resonant effect looms at medium to high speed. This is the phenomenon known as midfrequency or midrange instability. At higher speeds where the power amplifiers saturate, the damping ratio can fall to nearly zero or even become negative. The machine breaks into a growing oscillation that leads to loss of synchronism. A system that behaves this way must either be operated only at low speed or brought under control by means of a damping method.
Cures for resonance
As luck would have it, simply attaching a load to the step-motor shaft may provide some damping. Just adding inertia does not raise the damping ratio, but connecting inertia through a lossy coupling does. The backlash or play in gears and belt drives often helps damp the rotor.
To raise the damping ratio, engineers often resort to a viscous damper. This passive device attaches to a rear shaft extension. It consists of a so-called seismic mass coupled to the shaft through a viscous or visco-elastic medium. The device works well for achieving low speed smoothness (less than 3 revs/sec). Because it severely limits acceleration, it is not suitable for high-speed applications with rapid acceleration and deceleration profiles. Viscous dampers are also expensive, often costing nearly as much as the motor itself.
The modern solution for resonance is an antiresonant drive. With microstepping, an antiresonant drive can produce damping ratios as high as 0.7. This eliminates midrange instability as well as the ubiquitous ringing that threatens loss of synchronism.
These drives use sensorless feedback from observers embedded in the power amplifiers to provide damping information. The signal is applied to the command sequence to create a virtual viscous friction. Such friction opposes ringing without interfering with other move dynamics. As a result the engineer may plan on using close to 90% of the step motor's available torque.