Three-phase motors rarely get along with harmonics. In fact, excessive harmonics tend to act as a brake, reducing efficiency. Therefore, most design efforts have been directed at reducing, masking, or eliminating harmonics either at the point where utility power comes into a plant or where current comes into the motor.
But what if you wound the motor to increase the number of phases beyond three, say to 12, or even higher? Researchers have done that and discovered that instead of damping torque, the harmonics in ac inverter-based motors work with the phases to boost torque.
In the case of a proprietary, patentpending 18-phase prototype, there is 30% more continuous torque and at least three times as much peak torque as comparable three-phase ac motors. The researchers claim that this winding technique retrofits to existing ac units.
The engineers took a three-phase motor and increased the number of phases to the point where each polephase group consisted of only a single slot in the stator winding. This means that an ac motor can operate with upwards of twelve phases. If there's enough stator iron, twenty-four or more phases are possible. And with custom iron, there is no upper limit on phase count.
The factors behind the higher torque are best explained through copper use and harmonics, with harmonic waveforms divided into temporal waves in the power supply current, and spatial waves in the airgap flux.
Spin to harmony
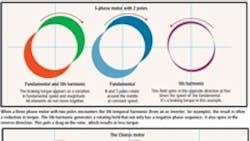
In a typical three-phase motor, the fundamental frequency of the applied current voltage generates the major rotating magnetic field and sets synchronous speed. Analysis shows that the magnetic field rotates at a speed determined by the applied frequency and the number of poles.
Each of the harmonics found in the current generates a rotating field as well. Each field is also subject to the same synchronous speed formula.
Now in a three-phase motor, the fundamental electrical angle between phases is 120°. But windings are on both sides of the stator, so the electrical phase angle between adjacent polephase groups is 60°.
For the fifth harmonic in the drive waveform, the electrical angle between phases is five times this, or 300°. Because sinusoids are periodic functions, 300° is the same as -60°; thus, the phase relation between adjacent phases for the fifth harmonic is precisely opposite that of the fundamental. Therefore, in a three-phase motor, the fifth temporal harmonic generates a rotating field with the same number of poles as that produced by the fundamental, but with negative phase sequence.
Additionally, the fifth harmonic has five times the frequency of the fundamental. Therefore, its rotating field spins at five times the fundamental speed in the reverse direction. This puts a drag on the rotor, which ultimately results in less torque.
What happens, though, if you increase the number of phases, say to five? The fundamental electrical angle between phases becomes 72°, and the angle between pole phase groups is 36°.
For the fifth harmonic, the electrical becomes 360°, and the electrical angle between adjacent pole-phase groups is 180°. Over one 360° cycle of fundamental current flow in the stator, the fifth harmonic will cycle five times.
In five-phase winding, the fifth harmonic current flow produces a tenpole rotating field, rather than a twopole. And it will have a synchronous speed that's the same as that of the fundamental.
Now consider a fifteen phase motor. The phase angle is 24°, with an electrical angle of 12° between adjacent pole-phase groups.
For the fifth harmonic, the electrical angle is 60°, the same as in a tenpole three-phase machine. In fact, a fifteen-phase two-pole winding, fed with fifth harmonic current alone, has exactly the same slot currents as a three-phase ten-pole winding.
In a fifteen-phase motor, then, the fifth temporal harmonic develops a rotating field with five times the poles of the fundamental rotating field. However, because of the fifth harmonic's higher frequency, this field spins at the same synchronous speed as the fundamental field.
Similar reasoning applies to all harmonics. Until the harmonic order is such that its phase angle is greater than 360°, its field will rotate in synchronism with the fundamental field.
Continue on page 2
The effect is analogous to the minimum sampling frequency needed to construct a reasonable facsimile of a signal. The stator current pattern is spatially sampled, with each slot corresponding to a sampling point. Any components of the current pattern that have a higher frequency than the sampling rate, or number of phases, will be aliased. But any components of the current pattern of low enough frequency will be correctly represented, and will rotate in synchronism with the fundamental.
Thus, in a three-phase motor, the fifth harmonic in the drive wave form has the appropriate phase relation to produce a ten-pole field. However, this field is aliased into a two-pole field of different rotational rate. In a five or greater phase motor, however, the fifth harmonic ten-pole field is correctly represented.
All temporal harmonics up to the number of phases will be co-opted to have the same synchronous speed as the fundamental.
The spatial side
The magnetic field of any motor phase has a spatial structure that may be analyzed by Fourier series into a set of sinusoidal wave functions. Each motor winding occupies a physical angle that determines the minimum angular change of the developed magnetic field.
In a three-phase motor, the angular difference between windings of the fundamental rotating field is 60°. For the fifth harmonic, this angular difference is 300°, or -60°. In this case, the fundamental applied voltage generates the fifth spatial harmonic, superimposed upon the fundamental, and the frequencies match. The harmonic's rotating field, though, has five times the pole count, and a synchronous speed one fifth that of the fundamental. Such spatial harmonics can cause dips in the torque-speed curve at low speeds, leading to cogging, dragging, or other torque irregularities.
As the number of phases increases, the electrical angle between phases decreases. In a five-phase machine, the angular difference between windings is 36°, and the fifth harmonic is no longer aliased. It will now rotate in synchronism with the fundamental rotating field, simply changing the shape of the field.
Similar arguments apply to higher order harmonics with increased phase counts. Again, spatial harmonics up to the number of phases will generate rotating fields of the same synchronous speed as the fundamental field.
Copper losses
Conventional three-phase motors usually use chorded and distributed windings to reduce the effects of spatial harmonics. Chorded windings do not span a full 180 electrical degrees. Therefore, the back emf voltages induced in each half winding are not in phase with each other, reducing the total induced voltage. Flux density will expand unless the number of winding turns is increased. The benefit is that the phase difference between the winding sides is different for different harmonics, weakening the harmonic fields relative to the fundamental field.
In distributed windings, the same electrical phase is applied to several windings at different electrical angles. This reduces the total voltage induced in the windings. Unless the number of turns is increased, flux density will again rise. But the distribution factor has greater effect on harmonics than on the fundamental, weakening the harmonic fields relative to the fundamental field.
Both chording and distribution increase the number of turns required to match a winding to a particular voltage. This generally increases the length of copper wire needed to form a winding. The wire also needs to fit into the stator slot, and so must be made thin. The net result is that chording and distribution both act to increase the resistance of the winding. This increases the winding resistance losses, but decreases spatial harmonic losses.
While winding distribution and chording reduce spatial harmonics, they can enhance sensitivity to temporal harmonics. Thus, the penalty for enhanced torque quality in conventional motors is decreased efficiency when these same motors are run with drive currents that include harmonic content.
However, a high-phase order motor, because it makes harmonic elimination unnecessary, can use concentrated full span windings with unity chording and distribution factors. Then, fewer turns are needed for a given voltage, reducing winding resistance. Effectively, the winding increases the apparent conductivity of the copper wire.
The result is a motor with enhanced efficiency in normal conditions and better overload capability. In addition, the motor works with smaller and simpler inverters.
Going into overdrive
Harmonic synchronism is valuable when the drive voltage has harmonic content. Perhaps more importantly, when the motor is driven to high levels of saturation, harmonic synchronization really shines.
Conventional high-efficiency motors keep saturation levels low to minimize spatial harmonics. Higher saturation levels, however, increase power handling capacity and are especially beneficial in starting overloads and intermittent overloads for both motors and generators. Advanced control systems for conventional three-phase motors take advantage of this by adjusting saturation levels to match load.
Continue on page 3
In any motor, iron permeability will decrease with increasing saturation, reducing inductance, which will increase current flow in the windings. More current will result in greater heat in the windings, which will reduce motor efficiency. In addition, the non-linear inductance will distort the magnetic field, which will affect the harmonic creation, and thus, motor efficiency.
In the Chorus prototype motor, however, while there are increased current losses as saturation increases, distortion-created harmonics have less effect here than on other designs. In a high polyphase motor like the Chorus, spatial harmonics are put to use and thus do not present a serious loss. Winding heating associated with the increased magnetizing current affects three-phase and polyphase motors the same.
Proper winding techniques on three-phase motors can reduce spatial harmonics effects. They tend to cancel spatial harmonics and cause motors to act as short circuits for temporal harmonics. For three-phase motors, the common practice is to derate power by 10% when operated with inverters. Because of their windings, high polyphase motors need not be de-rated. Therefore, available power handling is about 15 to 20% higher with the same motor heating.
In general, available rotor current scales linearly with saturation, and available torque scales as the square of the saturation. As applied drive voltage is increased, so do magnetic flux and available rotor current. For a given fixed power output, this means that rotor current decreases as applied drive voltage increases, but that more power output is available. Increases in drive voltage and saturation thus mean increased saturation related losses, but decreased rotor current related losses. For any given power output, there will be an ideal combination of drive voltage and current which will result in maximum efficiency for that output.
An overload situation can be defined as a motor operational state that produces torque or power output greater than the continuous rating of the motor, but which would result in motor overheating if maintained continuously. The extent of the overload can be described in terms of the time duration over which the overload can safely be maintained. Generally, the shorter the period of the overload, the greater the output level. The researches operated the polyphase Chorus prototype at overload levels for about 30 seconds, until it reached maximum temperature. The torque measured was three times the nominal breakaway torque of the original three-phase motor that had been rewound to build the prototype, implying a saturation increase of 73% over that seen in a three-phase motor at breakaway.
Out with the new, in with the old
By effectively using spatial harmonics, it's possible to use those higher saturation levels. Even though iron and eddy current losses rise at high saturation levels, the losses from harmonic current flows and conflicting rotating fields will greatly drop.
Higher harmonic tolerance also allows slower switching speeds in the drive electronics. Therefore, drives can use SCR and GTO devices rather than IGBTs. SCRs also offer an overload capacity of 6 to 10 sec, versus fractions of a second for IGBTs.
This development resurrects the ability to use square wave inverters. Square waves are rich in odd order harmonics. The drives that send out these waves usually use older technology, such as SCRs, and so are often simpler and less expensive to design and build than sine wave versions. Plus, the power electronics operate at lower frequencies and have fewer losses.
Beyond the fifth
There's nothing particularly special about the fifth harmonic in the fifteen-phase motor. It was chosen only because the phase angles correspond to those of the fundamental in a three-phase motor of higher pole count. But as long as the phase angle of the current feeding a particular winding matches the electrical angle of that winding, you have the conditions for harmonic torque boost.
In the case of a fifteen-phase, two-pole motor, windings are 12° apart. The currents feeding adjacent windings have a timing difference corresponding to 12° of an electrical cycle. This difference between the fifth harmonic waveforms is exactly the same as that of the fundamental, just at five times its frequency. When the difference is measured in terms of degrees, the phase angle between adjacent windings is 60°.
Consider a 10 Hz fundamental. Each cycle is 100 msec. Adjacent windings are driven 1/30th of a cycle apart, or 12°, for a time delay of 3.33 msec. The fifth harmonic is locked in time with the fundamental, so each winding is still driven with a 3.33 msec time delay. But the harmonic's period is 20 msec, so this delay corresponds to 1/6th of a cycle, or 60°. (Remember, in this fifteen-phase motor, each winding has two sides. You drive 15 phases, but get 15 more "for free" on the opposite coil sides for a total of 30 current phases.)
For the eleventh harmonic with a 12° fundamental time delay, adjacent windings are driven 132° apart, producing a 22-pole magnetic field structure. Divide the number of current phases by the pole count for a result of 16.364 slots per pole. This 22-pole rotating field spins in synchronism with the fundamental.
Now, look at an 11-phase motor. With 22 stator slots each winding is 16.364° apart. The fundamental phase difference is similarly 16.364°. For the eleventh harmonic, the phase difference is 180°, meaning that adjacent slots are energized in inverse relation. This is the limiting case of 1 slot per pole. For the eleventh harmonic, the currents flowing in the slots are exactly the same as those in a 22- pole single phase machine.
The synchronism fails at harmonics above the number of phases. Consider the 15-phase motor but with a 17th harmonic. The fundamental electrical angle is 12°, so the 17th harmonic electrical angle will be 204°. The electrical phase angle is now greater than 180° for this harmonic and the positive increase of 204° per winding corresponds to a negative value of 156°, which happens to be the same as the electrical angle for the thirteenth harmonic. Thus in a 15- phase motor, the 17th harmonic produces a negative phase sequence rotating field with the same pole count as the 13th harmonic. This is exactly the sort of aliasing that occurs in three-phase motors with harmonics above the third.
Chris Bourne is with Borealis Technical Limited, Middlesex, England.